(x y) 1 6?What is the coefficient of the x 3 y 13 x^{3}y^{13} x 3 y 1 3 term in the polynomial expansion of (x y) 16?X y is a binomial in which x and y are two terms In mathematics, the cube of sum of two terms is expressed as the cube of binomial x y It is read as x plus y whole cube It is mainly used in mathematics as a formula for expanding cube of sum of any two terms in their terms ( x y) 3 = x 3 y 3 3 x 2 y 3 x y 2

The Binomial Theorem Notes Answers Binomial Theorem Notes Ans3 3 Using Above Expansion X X Y X Y Xy Y X X Y X Y Xy X Y X Y Xy Y The Binomial Theorem Notes Answers Date Rhhs Pdf Document
X y 3 expand
X y 3 expand-(x y) 3 = x 3 3x 2 y 3xy 2 y 3 (x y) 4 = x 4 4x 3 y 6x 2 y 2 4xy 3 y 4;FAQs on (a b)^3 Formula What Is the Expansion of (a b) 3 Formula?



1
A Taylor Series is an expansion of some function into an infinite sum of terms, where each term has a larger exponent like x, x 2, x 3, etc Example The Taylor Series for e x e x = 1 x x 2 2!Precalculus Precalculus questions and answers 2) According to the Binomial Theorem, one of the terms in the expansion of (x y8 IS A) 8x B) 8y8 9 56x8y8 D) 56xy3Identifying Binomial Coefficients In Counting Principles, we studied combinationsIn the shortcut to findinglatex\,{\left(xy\right)}^{n},\,/latexwe will need to use combinations to find the coefficients that will appear in the expansion of the binomial
write down the first four terms of the binomial expansion of (1y)^8 in ascending power of yBy putting y=1/2x(1x) in your expansion, find the value of p and q if 11/2x(1x)^8=14xpx^2qx^3 Maths Find the first four terms in the binomial expansion of (1x)^(1/5) and state the range of values of x for which this expansion is validHenceX n 3!) 2 n1 (3) n2 (4) n3 x n1 y n2 z n3 Putting n1 = 3, n2 = 4, n3 = 27 What is the coefficient of x' in (2 x)19?
Example 3 In the expansion of (x − y) 15, calculate the coefficients of x 3 y 12 and x 2 y 13 Solution The coefficient of x 3 y 12 is positive because the exponent of y is even That coefficient is 15 C 12 But 15 C 12 = 15 C 3, and so we haveX^3 y^3 z^3 3x^2y 3xy^2 3x^2z 3z^2x 3y^2z 3z^2y 6xyz Lennox Obuong Algebra Student Email obuong3@aolcomThe (a b) 3 formula is also known as one of the important algebraic identities It is read as a minus b whole cube



What Is The Answer Of X Y Quora



Alg2 10 5 Part 2 Binomial Expansion Mrsb Schooltube Safe Video Sharing And Management For K12
Binomial Theroem 0 2299 6 535 Find the coefficient of x^3 y^3 z^2 in the expansion of (xyz)^8 MathCuber 0 users composing answersThe binomial expansion of a difference is as easy, just alternate the signs (x y) 3 = x 3 3x 2 y 3xy 2 y 3In general the expansion of the binomial (x y) n is given by the Binomial TheoremTheorem 671 The Binomial Theorem top Can you see just how this formula alternates the signs for the expansion of a difference?The following are algebraix expansion formulae of selected polynomials Square of summation (x y) 2 = x 2 2xy y 2 Square of difference (x y) 2 = x 2 2xy y 2 Difference of squares x 2 y 2 = (x y) (x y) Cube of summation (x y) 3 = x 3 3x 2 y 3xy 2 y 3 Summation of two cubes x 3 y 3 = (x y) (x 2 xy y 2) Cube




14 Pokemon Xy Flashfire Expansion Set Base 3 Butterfree




Expanding Binomials Video Series Khan Academy
Each term r in the expansion of (x y) n is given by C(n, r 1)x n(r1) y r1 Example Write out the expansion of (x y) 7 (x y) 7 = x 7 7x 6 y 21x 5 y 2 35x 4 y 3 35x 3 y 4 21x 2 y 5 7xy 6 y 7 When the terms of the binomial have coefficient(s), be sure to apply the exponents to these coefficients Example Write out theConsider power series expansion f (x)= X1 n=0 cn (x¡a) n =c 0c1(x¡a)c2(x¡a) 2c 3(x¡a) 3 (3) for general function f (x)about x =a Setting x =a;The x^2 term is the rightmost one here so we'll get 1 times the first term to the 0 power times the second term squared or 1*1^0* (x/5)^2 = x^2/25 so not here 1 3 3 1 for n = 3 Squared term is second from the right, so we get 3*1^1* (x/5)^2 = 3x^2/25 so not here 1 4 6 4 1 for n = 4



1




Expand 1 X Y 3 3 Solve It Fastly Brainly In
The coefficient of x 3 y 4 z 5 in the expansion xy yz xz 6 isA 40В 70C 50D 60 We know that General term of expansion (a b)n is Tr1 = nCr an–r br For (x 2y)9, Putting n = 9 , a = x , b = 2y Tr 1 = 9Cr (x)9 – r (2y)r = 9Cr (x)9 – r (y)r (2)r We need to find coefficient of x6 y3 Comparing yr = y3 r = 3 Putting r = 3 in (1) T31 = 9C3 x9 – 3 y3Rearranging the terms in the expansion, we will get our identity for x 3 y 3 Thus, we have verified our identity mathematically Again, if we replace x with − y in the expression, we have




Amazon Com Pokemon Beedrill Kaukuna And Weedle Rare Card Evolution Set Xy 3 4 And 5 146 Toys Games




Tronxy Xy 3 Pro Desktop 3d Printer Kit 300 300 400mm With 3 5 Inch Full Color Touchscreen Extruder Silent Motherboard 3d Printer 3d Printers Aliexpress
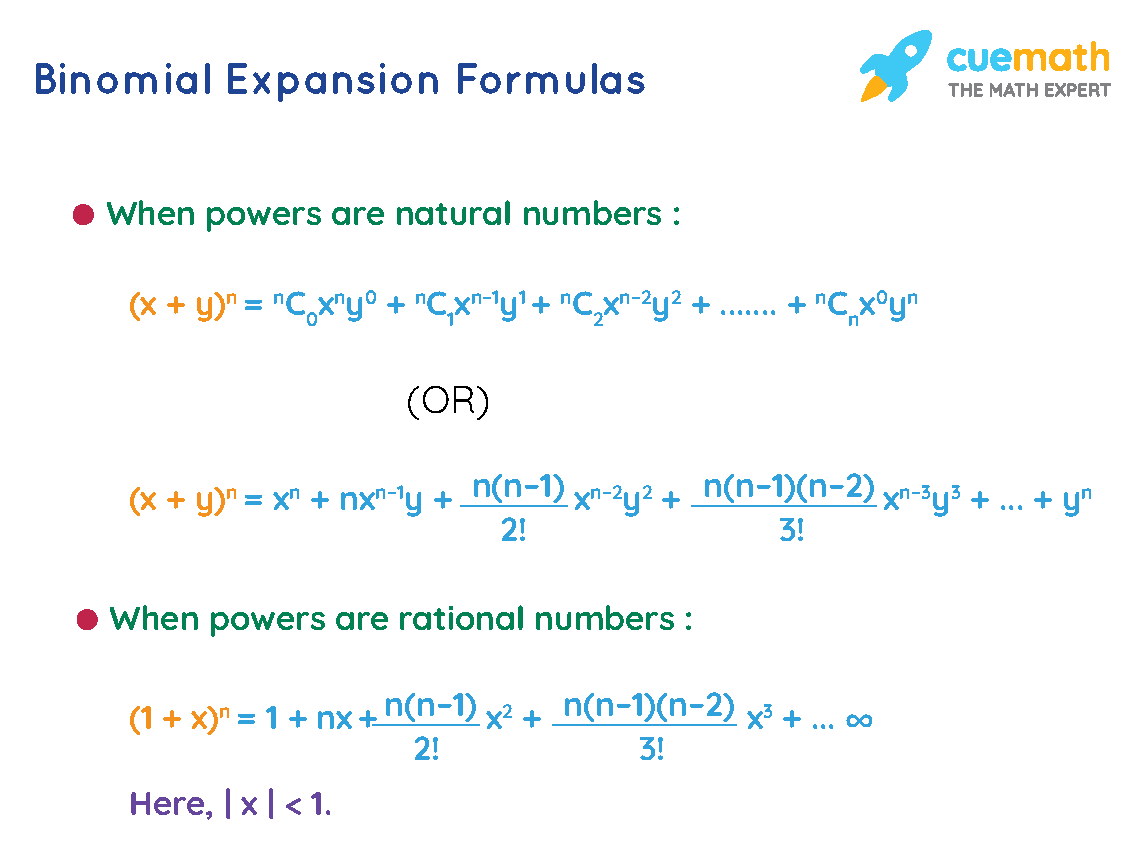
If a binomial expression (x y) n is to be expanded, a binomial expansion formula can be used to express this in terms of the simpler expressions of the form ax by c in which 'b' and 'c' are non negative integers The value of 'a' completely depends on the value of 'n' and 'b'We obtain f (a)=c0 Next, we take derivative on (3) so that f 0(x)= X1 n=1 cnn(x¡a) n¡1=c 1c2¢2(x¡a)c3¢3(x¡a) 2c 4¢4(x¡a) 3 (4) Setting xIt is quite easy if you know Pascal's triangle Pascal's triangle represents coefficients of binomial expansion with inegral index 3rd term will be 15a⁴b², and its coefficient is 15 You can also make use of general term which is represented by T (r1) and given as, T (r1)= nCr a^ (nr)b^r In your case n=6, and for 3rd term put r=2
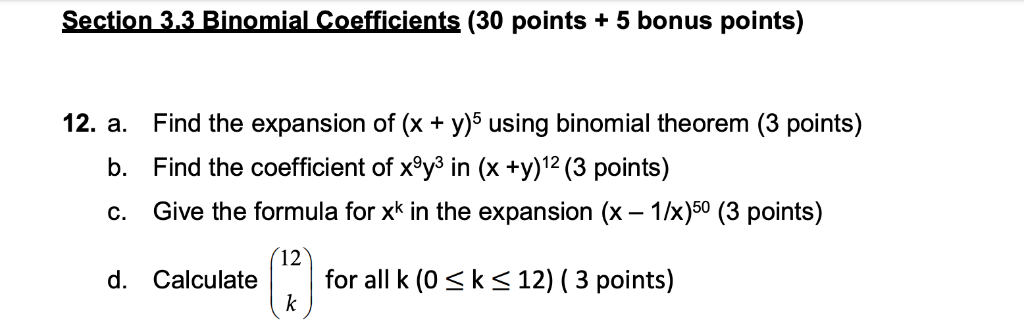



Solved Section 3 3 Binomial Coefficients 30 Points 5 Bonus Chegg Com




Binomial Theorem Wikipedia
8 What is the coefficient of r8yº in the expansion of (3x 2y)17Key Takeaways Key Points According to the theorem, it is possible to expand the power latex(x y)^n/latex into a sum involving terms of the form latexax^by^c/latex, where the exponents latexb/latex and latexc/latex are nonnegative integers with latexbc=n/latex, and the coefficient latexa/latex of each term is a specific positive integer depending on latexn/latexExpand (xy)^3 (x y)3 ( x y) 3 Use the Binomial Theorem x3 3x2y3xy2 y3 x 3 3 x 2 y 3 x y 2 y 3



Ex 2 The 5th Term In The Expansion Of Frac 2x 3 Frac 3 2x 6 Is A 45x 2 B Frac 45 2 X 3 C Frac 135 4 X 3 Frac 135 4 X 2 2x 6 R 3y5 Snapsolve




Exponent Rules 7 Key Strategies To Solve Tough Equations Prodigy Education
( n − k)!To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW Find the coefficient of `x^2 y^3 z^4` in the expansion of ` (axbycz)^9`Solution The expansion is given by the following formula ( a b) n = ∑ k = 0 n ( n k) a n − k b k, where ( n k) = n!




Using Binomial Theorem Expand X Y 5 X Y And Hence Find The Value Of Root 2 1 5 Root 2 1 5 Maths Binomial Theorem Meritnation Com




View Question What Is The Coefficient Of The X7y3 Term In The Expansion Of X Y 10
My first and naive impression is that the result is 0 but according to Salinas, Introduction to Statistical Physics that's $3x^{1/2}y O(x/y)^3$ I think Taylor expansion would do it The thingFind the coefficient of x 3 y 4 z 2 in the expansion of (2x – 3y 4z) 9 Sol General Term in (2x – 3y 4z) 9 = 9!X n 3!) (2x) n1 (3y) n2 (4z) n3 = 9!



Alg2 March28 The Answers




Binomial Expansion Essentials For Act Act Math Pascal S Triangle Acting
Binomial Theorem Formula When the number of terms is odd, then there is a middle term in the expansion in which the exponents of a and b are the same Only in (a) and (d), there are terms in which the exponents of the factors are the sameAlthough the formula above is only applicable for binomials raised to an integer power, a similar strategy can be applied to find the coefficients of any linear polynomial raised to an integer power Preexpansion, there are $8$ factors of $2x y 5$ From those $8$ factors, choose the $3$ that contribute to the $x^3$, from the remaining $5$ factors, choose the




15 Pokemon Xy Roaring Skies Expansion Set Reverse Foil Wurmple 3 M0p Ebay
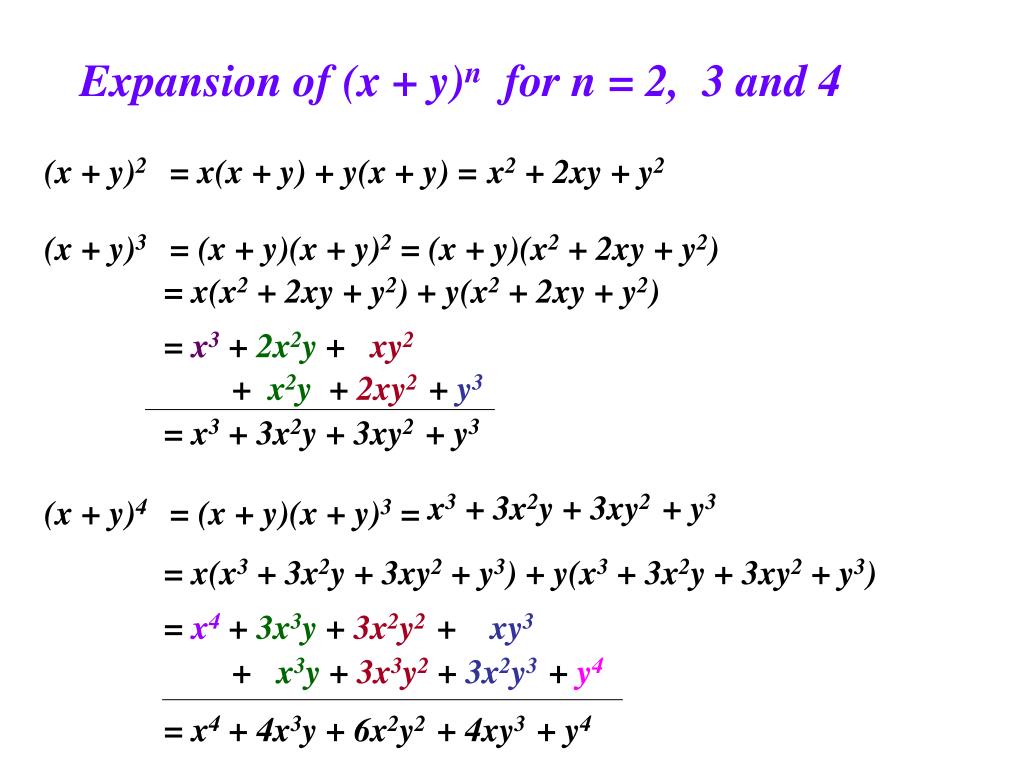



Ppt The Binomial Theorem 1 Powerpoint Presentation Free Download Id
Free expand & simplify calculator Expand and simplify equations stepbystepBinomial Expansions Binomial Expansions Notice that (x y) 0 = 1 (x y) 2 = x 2 2xy y 2 (x y) 3 = x 3 3x 3 y 3xy 2 y 3 (x y) 4 = x 4 4x 3 y 6x 2 y 2 4xy 3 y 4 Notice that the powers are descending in x and ascending in yAlthough FOILing is one way to solve these problems, there is a much easier wayGet stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!
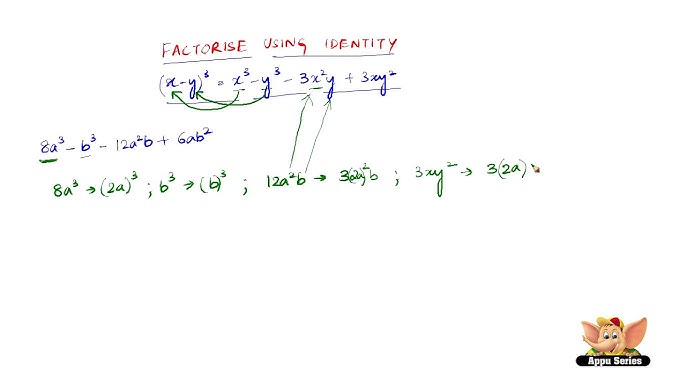



How To Expand Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube




Amazon Com Evolutions Pokemon Tcg Xy 3 Card Pack Sealed Official Mini Booster Toys Games
The exponential function is a mathematical function denoted by () = or (where the argument x is written as an exponent)It can be defined in several equivalent waysIts ubiquitous occurrence in pure and applied mathematics has led mathematician W Rudin to opine that the exponential function is "the most important function in mathematics" Its value at 1, = (), is a mathematicalQuestion 3 Find the expansion of (x y)6 4 Find the coefficient of x5 y8 in (x y)13 5 How many terms are there in the expansion of (x y)100 after like terms are collected?Free math lessons and math homework help from basic math to algebra, geometry and beyond Students, teachers, parents, and everyone can find solutions to their math problems instantly




Example 7 Find Coefficient Of X6y3 In Expansion X 2y 9




How Do You Expand The Binomial X Y 5 Socratic
In elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomialAccording to the theorem, it is possible to expand the polynomial (x y) n into a sum involving terms of the form ax b y c, where the exponents b and c are nonnegative integers with b c = n, and the coefficient a of each term is a specific positiveFactor x^3y^3 x3 − y3 x 3 y 3 Since both terms are perfect cubes, factor using the difference of cubes formula, a3 −b3 = (a−b)(a2 abb2) a 3 b 3 = ( a b) ( a 2 a b b 2) where a = x a = x and b = y b = y (x−y)(x2 xyy2) ( x y) ( x 2 x y y 2) Explanation (x −y)3 = (x − y)(x −y)(x −y) Expand the first two brackets (x −y)(x − y) = x2 −xy −xy y2 ⇒ x2 y2 − 2xy Multiply the result by the last two brackets (x2 y2 −2xy)(x − y) = x3 − x2y xy2 − y3 −2x2y 2xy2 ⇒ x3 −y3 − 3x2y 3xy2 Always expand each term in the bracket by all the other



Binomial Expansion Formulas Derivation Examples




Japanese Pokemon Xy Cp6 Expansion Pack th Anniversary Special Pack Japanese Pokemon Products Japanese Starters Decks Gift Boxes Collector S Cache
6 What is the coefficient of x7 in (1 x)11?An outline of Isaac Newton's original discovery of the generalized binomial theorem Many thanks to Rob Thomasson, Skip Franklin, and Jay Gittings for their243x 5 810x 4 y 1080x 3 y 2 7x 2 y 3 240xy 4 32y 5 Finding the k th term Find the 9th term in the expansion of (x2y) 13 Since we start counting with 0, the 9th term is actually going to be when k=8 That is, the power on the x will 138=5 and the power on the 2y will be 8



How Do You Use The Binomial Theorem To Expand X Y 5 Socratic




Pokemon Xy Furious Fists Xy3 Lot Of 5 Booster Packs
Find the 6th term of the expansion (y^1/2 x^1/3)^n , if the binomial coefficient of the 3rd term from the end is 45 asked Jul 28 in Binomial Theorem by Kanishk01 ( 459k points) binomial theoremThis has both positive and negative terms, so it can be compared with the expansion of (x − y) 3 The terms of polynomials are rearranged Then terms that are perfect cubes are identified Comparing the polynomial with the identity we have, x = 2 a & y = 3 b(a b) 3 formula is read as a minus b whole cube Its expansion is expressed as (a b) 3 = a 3 3a 2 b 3ab 2 b 3 What Is the (a b) 3 Formula in Algebra?



Solved Coefficient Of Themal Expansion Versus Temperature Chegg Com



Tutorial 54 The Binomial Theorem
The Binomial Theorem is the method of expanding an expression which has been raised to any finite power A binomial Theorem is a powerful tool of expansion, which has application in Algebra, probability, etc Binomial Expression A binomial expression is an algebraic expression which contains two dissimilar terms Ex a b, a 3 b 3, etcExpand the following product (3 x 1) (2 x 4) `(3x1)(2x4)` returns `3*x*2*x3*x*42*x4` Expand this algebraic expression `(x2)^3` returns `2^33*x*2^23*2*x^2x^3` Note that the result is not returned as the simplest expression in order to be able to follow the steps of calculations To simplify the results, simply use the reduce functionThe whole amount of terms in the expansion of (x y) n are (n 1) The summation of exponents of x and y is always n Binomial coefficients are known as nC 0, nC 1, nC 2,up to n C n, and similarly signified by C 0, C 1, C2, , C n




16 Pokemon Xy Evolutions th Anniversary Expansion Pack Base 3 Caterpie



Illustration Of 3 D Decap Allocation A 3 D Placement B Download Scientific Diagram
Utilize the Binomial Expansion Calculator and enter your input term in the input field ie, ( x y) 6 & press the calculate button to get the result ie, x 6 6 x 5 y 15 x 4 y 2 x 3 y 3 15 x 2 y 4 6 x y 5 y 6 along with a detailed solution in a fraction of seconds Ex (x1)^2 (or) (x7)^7 (or) (x3= 1 ⋅ 2 ⋅ ⋅ n We have that a = 2 x, b = 5, and n = 3 Therefore, ( 2 x 5) 3 = ∑ k = 0 3 ( 3 k) ( 2 x) 3 − k 5 k Now, calculate the product for every value of k from 0 to 3 Thus, ( 2Answer (1 of 5) First of all, we observe the following formula {{\left( a\,\,b \right)}^{\,3}}\,=\,{{a}^{\,3}}\,\,{{b}^{\,3}}\,\,3\,a\,b\,\left( a\,\,b \right
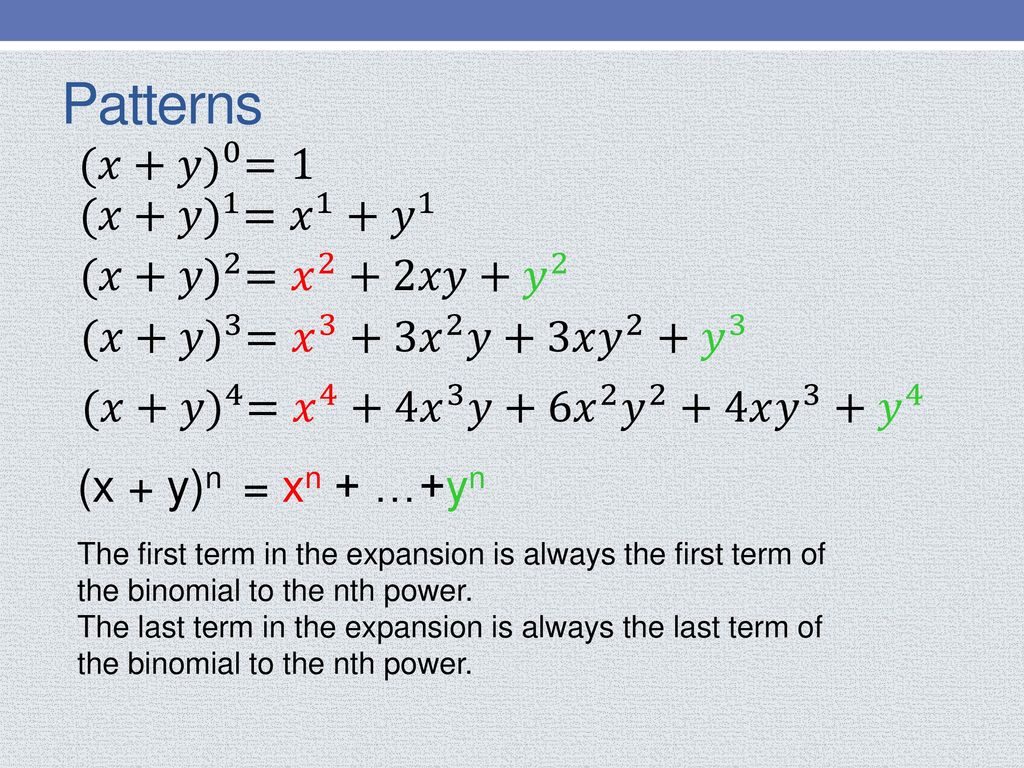



A Quick And Efficient Way To Expand Binomials Ppt Download
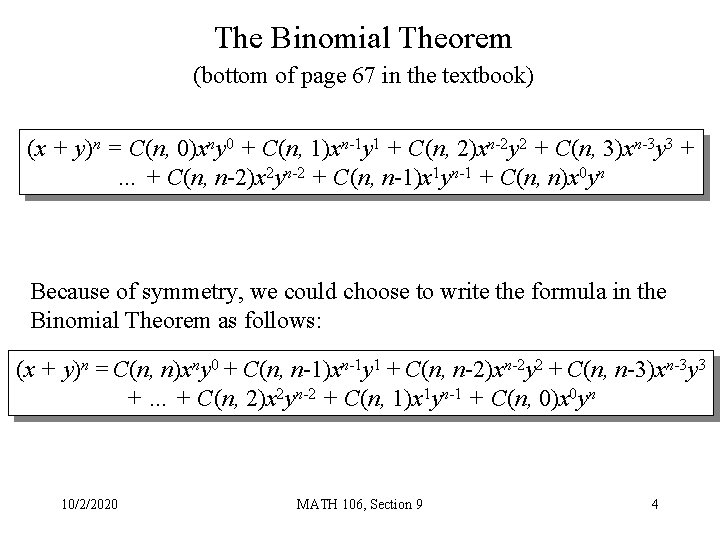



Section 9 Binomial Expansion Questions About Homework Submit



2




Binomial Expansion Christober S Technical Weblog



Print Tolerance Tuning Horizontal Xy Expansion Duet3d




Pokemon Tcg Xy Breakpoint Expansion Revealed Gotgame




Learn Algebraic Identity Of X Y And X Y In 3 Minutes



How To Expand The Binomial X Y 10 Quora




Learn Algebraic Identity Of X Y And X Y In 3 Minutes
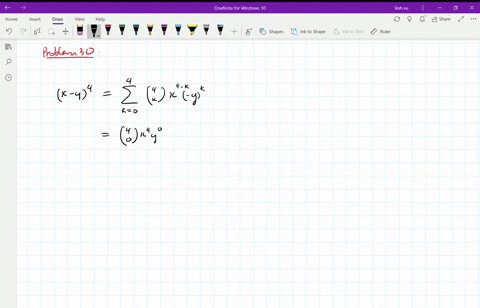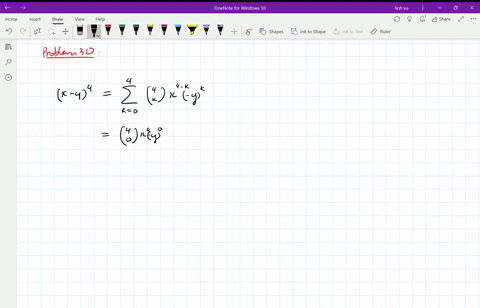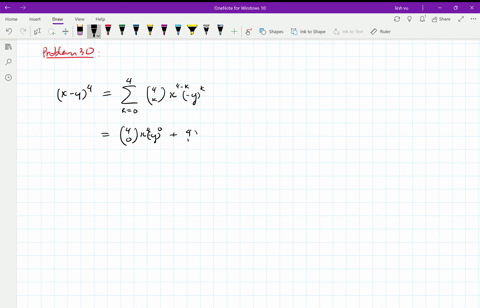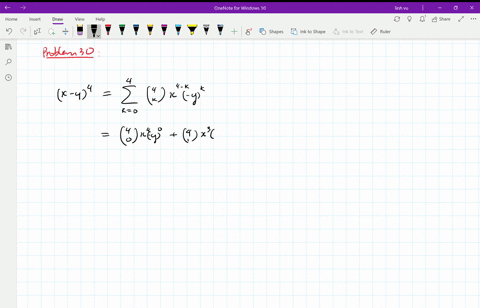



Solved Find The Expansion Of X Y 4 A Using Combinatorial Reasoning As In Example 1 B Using The Binomial Theorem




Gyarados Xy Expansion 3 Pack Blister Pokemon Trading Card Game Pokemon Trading Card Trading Cards Game Pokemon




Example 7 Find Coefficient Of X6y3 In Expansion X 2y 9




Learn Algebraic Identity Of X Y And X Y In 3 Minutes




The Binomial Theorem Notes Answers Binomial Theorem Notes Ans3 3 Using Above Expansion X X Y X Y Xy Y X X Y X Y Xy X Y X Y Xy Y The Binomial Theorem Notes Answers Date Rhhs Pdf Document




2 Use Eigenfunction Expansion To Solve The Following Ibvp U X T X T T 1 Sin M 0 0 Homeworklib



Expansion And Factorisation Bingo Card
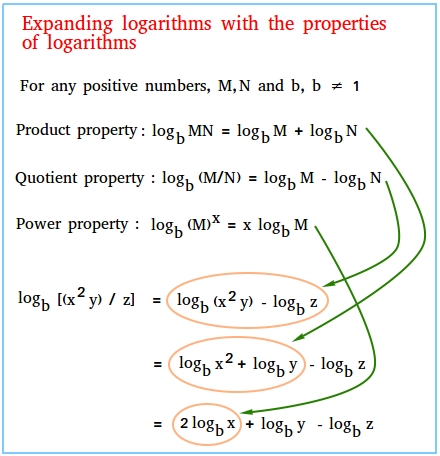



Expanding Logarithms




Binomial Expansion To Find A Specific Term Coefficient Mathematics Stack Exchange




Ml Aggarwal Solutions For Class 9 Chapter 3 Expansions Download Free Pdf




Assignment 5 Man 001 Indian Institute Of Technology Roorkee Man 001 Mathematics 1 B Tech I Studocu
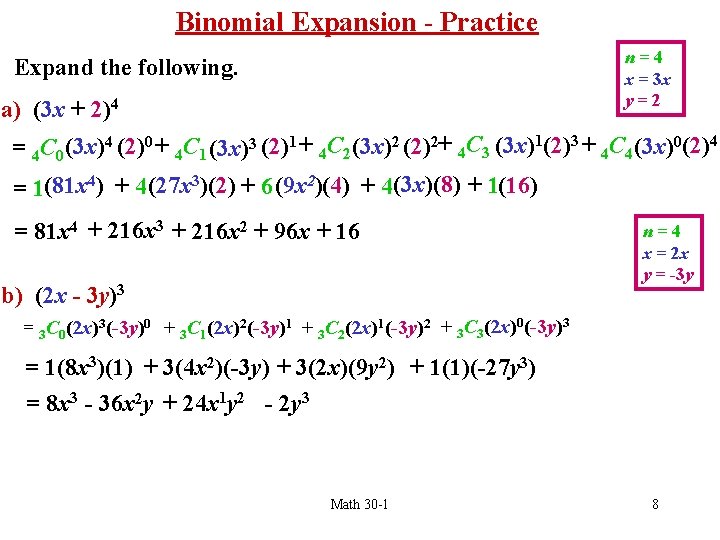



Myriorama Cards Were Invented In France Around 13



Expand 1 X Y 3 Whole Cube Studyrankersonline




Learn Algebraic Identity Of X Y And X Y In 3 Minutes



1
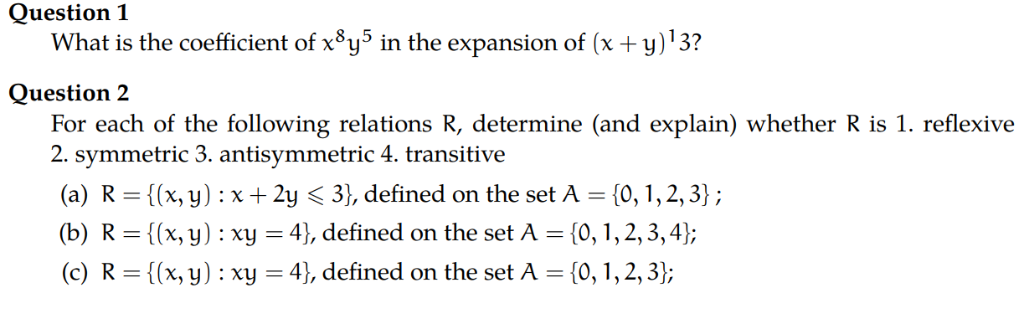



Solved Question 1 What Is The Coefficient Of X8y5 In The Chegg Com




Adaptive Infill Inner Horizontal Expansion Ideamaker Term Dictionary



2




Answered Consider The Following Min Term Bartleby
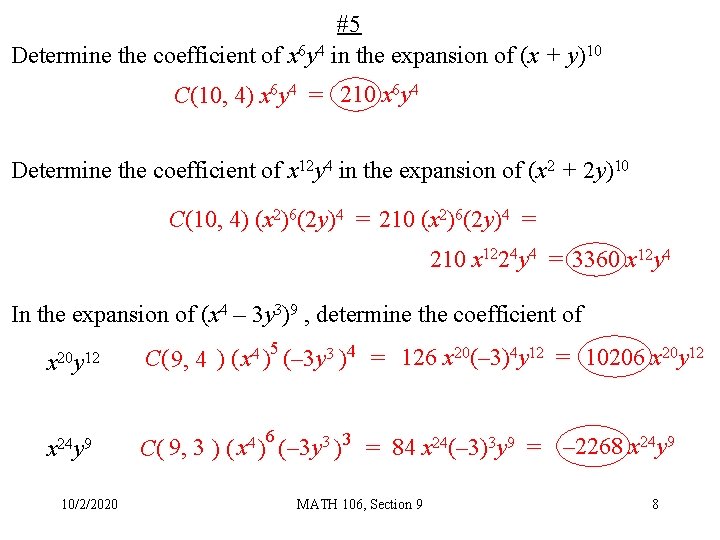



Section 9 Binomial Expansion Questions About Homework Submit




Sswlsr318cp Can Use The Binomial Theorem To Expand Any Power Of A Binomial Expansion Nco X




Pokemon Tcg Xy Expansion Phantom Forces Out Now




Expand X Y 3 And X Y 2 Brainly In




Physics Intuitions Geometric Representations Of Higher Degree Binomials




Section 8 5 The Binomial Theorem Ppt Download




The Binomial Theorem Kuta Software




Binomial Coefficients And The Binomial Theorem



Expand 3x Y 5 Using The Binomial Theorem Mathsgee Q A Network
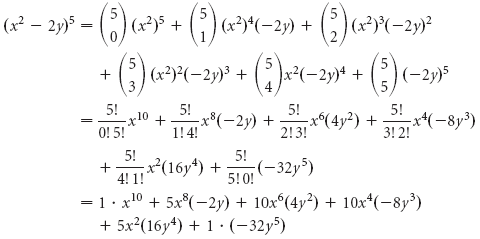



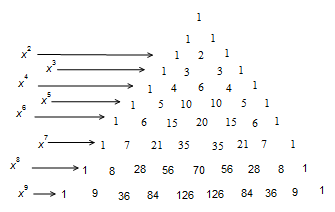
The Binomial Theorem Binomial Expansions Using Pascal S Triangle Subsets




Pokemon Tcg Xy Steam Siege 3 Booster Packs Coin Azelf Promo Card Pokemon Center Canada Official Site



The Binomial Expansion Of X Y22 Is A X2 2xy2 Y4 Gauthmath



Factorials To Binomial Theorem




16 Pokemon Xy Generations Expansion Set Caterpie 3 5e9 Ebay




How To Expand Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube




Binomial Expansion Theorem Notes Guided Examples Practice X Y Tpt




X Y 2 3 Find The Expansion Of The Following Brainly In




Expand And Simplify Binomial Squares 2x 3y 2 Youtube




Find The Coefficient Of X 6y 3in The Expansion Of X 2y 9



1




Themathbooklets S2t3 Expansion Of Linear Expressions




Write Down The Expansion By The Binomial Theorem Of 3 X Y 2 To The Power Of Maths Binomial Theorem Meritnation Com



How To Expand X Y 7 Quora




12 Expansion Of X Y 3 Y Z 3 Z X 3 Is A 2x 3 2y




Pokemon Announces Latest And Last Tcg Expansion For Xy




Tim Millett Tim Twmillett Twitter




Pokemon Tcg Xy Furious Fists Expansion Pocketmonsters Net



How Do You Use The Binomial Series To Expand 2x Y 9 Socratic
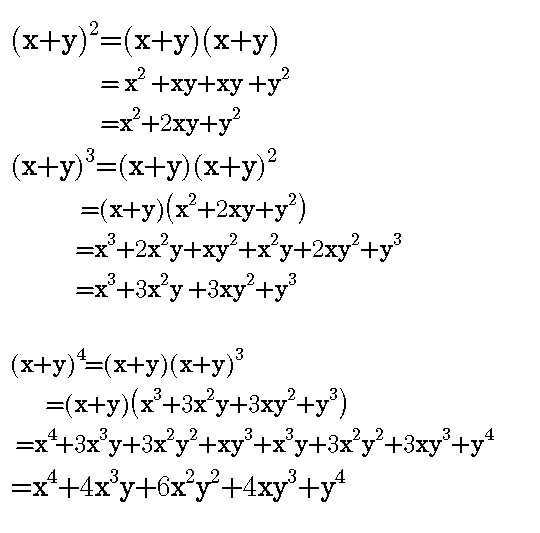



Worked Examples On Binomial Expansion Steemit




The Binomial Theorem Notes Answers Binomial Theorem Notes Ans3 3 Using Above Expansion X X Y X Y Xy Y X X Y X Y Xy X Y X Y Xy Y The Binomial Theorem Notes Answers Date Rhhs Pdf Document




Online Tutoring On Maths Binomial Theorem




Lesson Worksheet Pascal S Triangle And The Binomial Theorem Nagwa
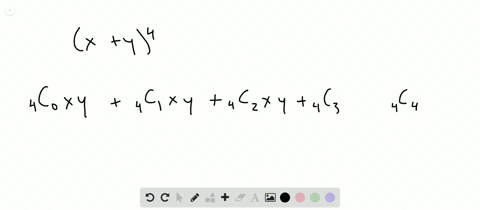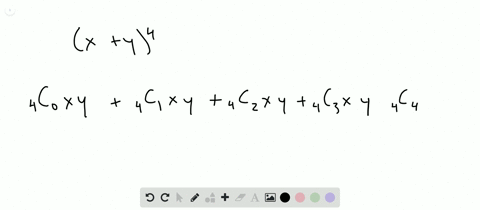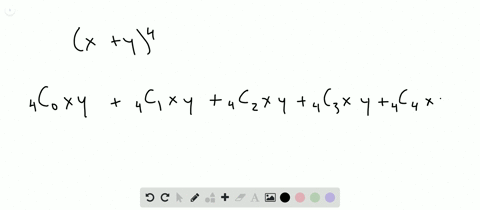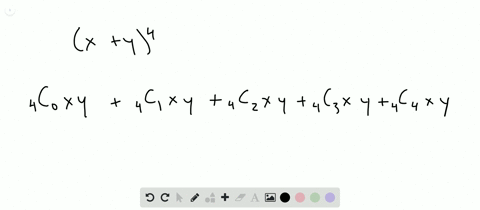



Solved Find The Expansion Of X Y 4 A Using Combinatorial Reasoning As In Example 1 B Using The Binomial Theorem




Using The Binomial Theorem College Algebra



Find The Coefficient Of The Term X 6y 3 In The Expansion Of X 2y 9 Sarthaks Econnect Largest Online Education Community




How To Expand Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube



Expand X Y 3 Sarthaks Econnect Largest Online Education Community
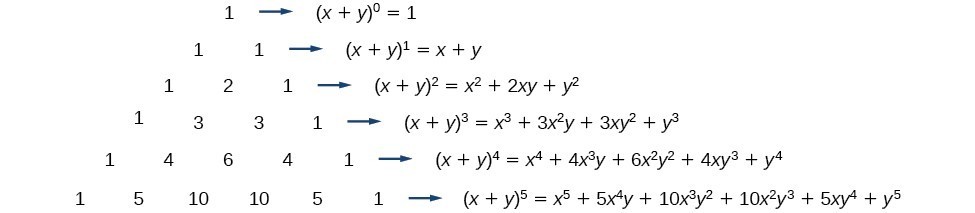



Using The Binomial Theorem College Algebra
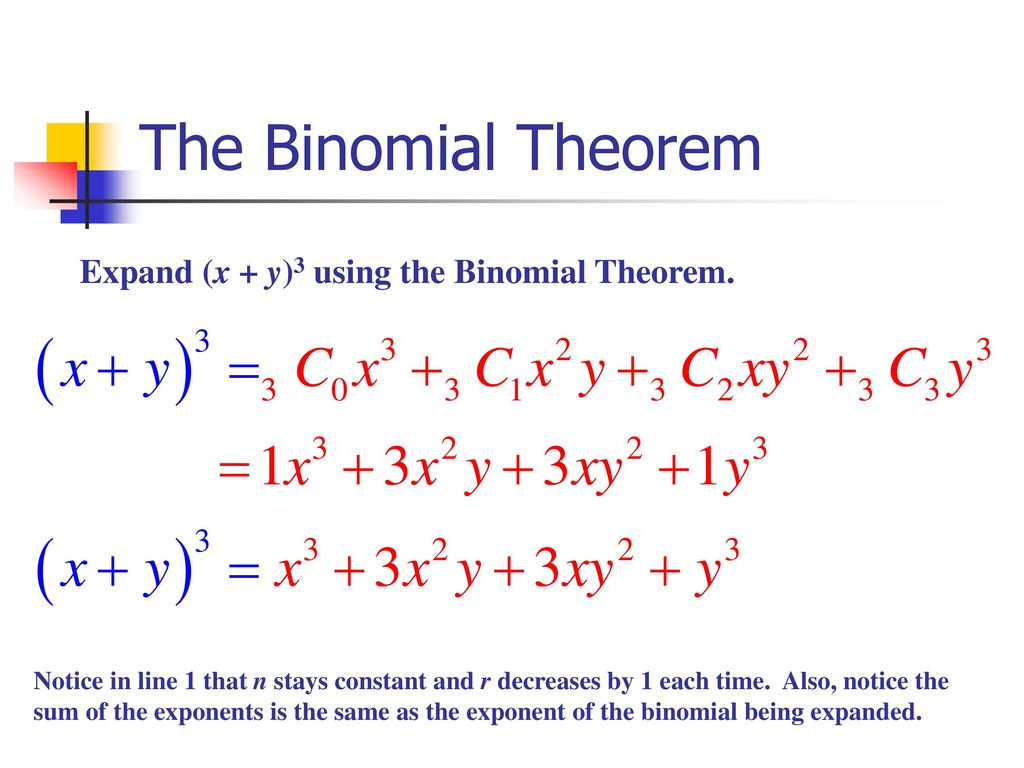



Section 8 5 The Binomial Theorem Ppt Download
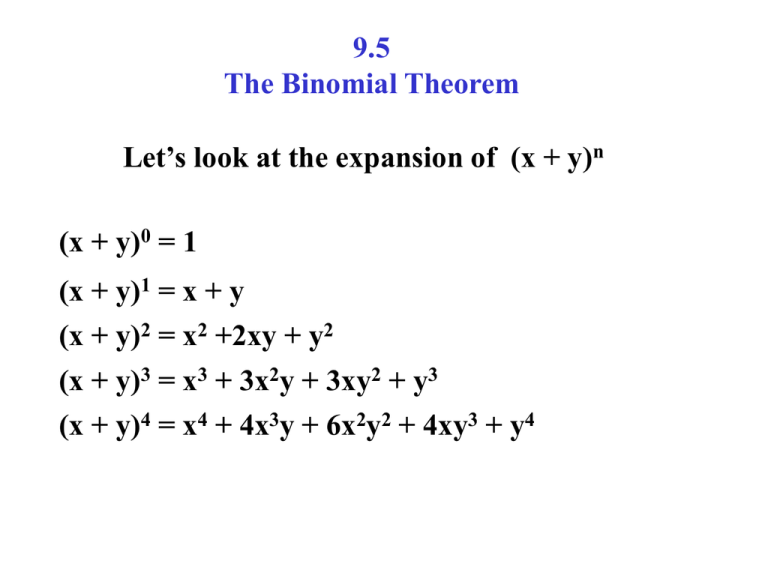



9 5 The Binomial Theorem



4 The Binomial Theorem
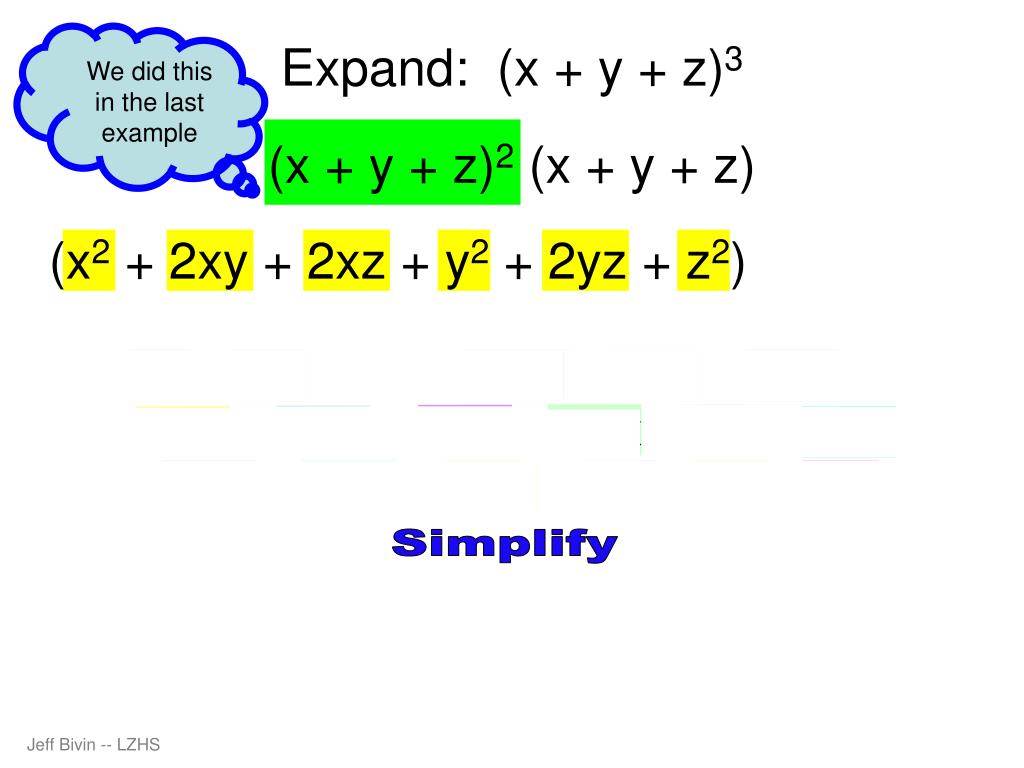



Ppt Binomial Expansion And More Powerpoint Presentation Free Download Id



Solved Find The 5th Term In The Expansion 3x 2y 6 2 What Is The Sum Of The First 36 Terms In The Arithmetic Series 0 2 0 Course Hero



Www Users Med Cornell Edu




Pok Eacute Mon Xy 12 Evolutions 3 Pack Walmart Com



1



0 件のコメント:
コメントを投稿